Recently, a customer asked how required quantity is calculated when using a scrap factor (%). Here's the scenario:
You have two components in the shop order (lot size = 1), but UOM in YK-S-P1 is PCS and in YK-S-P2 it is pcs (decimal allowed, qty calc = 2).
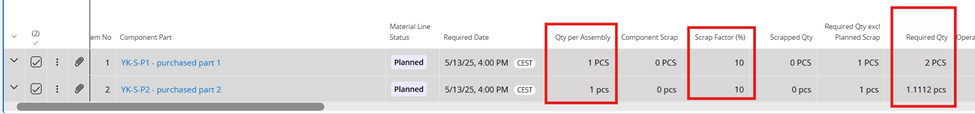
The problem customer had, why the required qty is not calculated as: (qty per assembly * scrap factor + qty per assembly)
According to that, for the second part required qty = 1 × 0.1 + 1 = 1.1. He asked why it was 1.1112?
The calculation here is done according to the equation: lot size/ (1-scrap factor)
So, for the first part: 1/ (1-0.1) = 1.1112 but the qty UOM here is PCS(decimals not allowed). Therefore, required qty = 2.
For the second part, the same calculation, since the decimals are allowed, qty required = 1.1112.
What is this equation lot size/ (1-scrap factor) and from where does it come from?
There are 2 ways you can derive this equation:
- Using Proportional Inverse Relationship
- Using Recursive Loss / Geometric Series (found this thanks to @sdhalk)
I have described both methods in the attached document below.
Why the customer’s logic was wrong? (qty per assembly * scrap factor + qty per assembly)
This approach incorrectly adds the scrap loss per total quantity to the per-unit quantity, which does not scale properly with the desired lot size.


